Trigonometric Identities – Problems

Trigonometric Identities – Problems

Trigonometric identities that involve trigonometric function that are true for every single value of the occurring variable. in other words, they are equation that hold true regardless of the value of the angles being chosen.

Trigonometric identities are as follow.

sin²θ + cos²θ = 1

sec²θ – tan²θ = 1

cosec²θ – tan²θ = 1

Example 1: Show that 2 (sin⁶x + cos⁶x) – 3 (sin⁴x + cos⁴x) + 1 = 0.

Solution: Given that 2 (sin⁶x + cos⁶x) – 3 (sin⁴x + cos⁴x) + 1

= 2 [(sin²x)³ + (cos²x)³] – 3 (sin⁴x + cos⁴x) + 1

= 2 [(sin²x + cos²x)³ – 3 sin²x cos²x (sin²x + cos²x)] [(sin²x + cos²x)² – 2 sin²x cos²x] + 1

= 2 (1 – 3 sin²x cos²x) – 3 (1 – 2 sin²x cos²x) + 1

= 0.

Hence proved.

Example 2: If tanθ + secθ = 3/2, Find sinθ, tanθ and secθ.

Solution: Given that tanθ + secθ = 3/2 … (1)

\(\sec \theta -\tan \theta =\frac{1}{\sec \theta +\tan \theta }=\frac{1}{\frac{3}{2}}\),

sec θ – tan θ = 2/3 … (2)

Solving equation (1) and (2)

sec θ + tan θ = 3/2

sec θ – tan θ = 2/3

—————————-

2 sec θ         = 13/6

—————————-

sec θ = 13/ 12

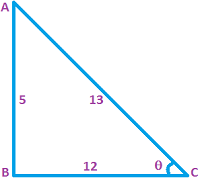
AC² = AB² + BC²

AB² = BC² – AC²

AB² = 5

From image

Tanθ = AB/ BC

= 5/ 12

Sinθ = AB/ AC

= 5/13.