Series Combination of Metallic Rods
1) Series Combination: Let n slabs each of cross sectional area\(A\), lengths \({{l}_{1}},\,{{l}_{2}},\,{{l}_{3}},\,{{l}_{4}}……{{l}_{n}}\) and conductivities \({{K}_{1}},\,{{K}_{2}},\,{{K}_{3}},\,{{K}_{4}}…..{{K}_{n}}\) respectively be connected in the series.
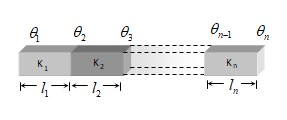
i) Heat Current: Heat current is the same in all the conductors. i.e., \(\frac{Q}{t}={{H}_{1}}={{H}_{2}}={{H}_{3}}=….={{H}_{n}}\)
\(\frac{{{K}_{1}}A\left( {{\theta }_{1}}-{{\theta }_{2}} \right)}{{{l}_{1}}}=\frac{{{K}_{2}}A\left( {{\theta }_{2}}-{{\theta }_{3}} \right)}{{{l}_{2}}}=\frac{{{K}_{n}}A\left( {{\theta }_{n-1}}-{{\theta }_{n}} \right)}{{{l}_{n}}}\),
ii) Equivalent thermal resistance: \(R={{R}_{1}}+{{R}_{2}}+{{R}_{3}}+{{R}_{4}}+….{{R}_{n}}\),
iii) Equivalent thermal conductivity: It can be calculated as follows, from \({{R}_{S}}={{R}_{1}}+{{R}_{2}}+{{R}_{3}}+….\),
\(\frac{{{l}_{1}}+{{l}_{2}}+…+{{l}_{n}}}{{{K}_{s}}}=\frac{{{l}_{1}}}{{{K}_{1}}A}+\frac{{{l}_{2}}}{{{K}_{2}}A}+….+\frac{{{l}_{n}}}{{{K}_{n}}A}\) \(\Rightarrow {{K}_{S}}=\frac{{{l}_{1}}+{{l}_{2}}+…+{{l}_{n}}}{\frac{{{l}_{1}}}{{{K}_{1}}}+\frac{{{l}_{2}}}{{{K}_{2}}}+…..+\frac{{{l}_{n}}}{{{K}_{n}}}}\),
a) For n slabs of equal length, \({{K}_{S}}=\frac{n}{\frac{1}{{{K}_{1}}}+\frac{1}{{{K}_{2}}}+…..+\frac{1}{{{K}_{n}}}}\),
b) For two slabs of equal length, \({{K}_{S}}=\frac{2{{K}_{1}}{{K}_{2}}}{{{K}_{1}}+{{K}_{2}}}\),
iv) Temperature of interference of composite bar: Let the two bars are arranged in series as shown in the figure
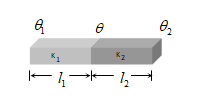
Then heat current is same in the two conductors. i.e.,
\(\frac{Q}{t}=\frac{{{K}_{1}}A\left( {{\theta }_{1}}-\theta \right)}{{{l}_{1}}}=\frac{{{K}_{2}}A\left( {{\theta }_{1}}-\theta \right)}{{{l}_{2}}}\),
By solving we get, \(\theta =\frac{\frac{{{K}_{1}}}{{{l}_{1}}}{{\theta }_{1}}+\frac{{{K}_{2}}}{{{l}_{2}}}{{\theta }_{2}}}{\frac{{{K}_{1}}}{{{l}_{1}}}+\frac{{{K}_{2}}}{{{l}_{2}}}}\),
(a) If \({{l}_{1}}={{l}_{2}}\) then \(\theta =\frac{{{K}_{1}}{{\theta }_{1}}+{{K}_{2}}{{\theta }_{2}}}{{{K}_{1}}+{{K}_{2}}}\),
(b) If \({{K}_{1}}={{K}_{2}}\) and \({{l}_{1}}={{l}_{2}}\) then \(\theta =\frac{{{\theta }_{1}}+{{\theta }_{2}}}{2}\).
