Removable Discontinuity
\(\underset{x\to a}{\mathop{\lim }}\,f(x)\) necessarily exists, but is either not equal to f(a) or f(a) is not defined. In this case, it is, possible to redefine the function in such that a manner that \(\underset{x\to a}{\mathop{\lim }}\,f(x)=f(a)\) and thus, make the function continuous.
Consider the function g(x) = (sin x)/x. the function is not defined at x = 0. So, the domain is R – {0}.
Since the limit of g at 0 is 1, g can be extended continuously to R by defining its value at 0 to be 1.
Thus, redefined function.
\(g(x)=\left\{ \begin{align} & \frac{\sin x}{x},\ \ x\ne 0 \\ & 1,\ \ \ \ \ \ \ \ x=0 \\\end{align} \right\}\) is continuous at x = 0.
Thus, a point in the domain that can be filled in so that the resulting function is continuous is called a removable discontinuity.
Example: Find the Removable Discontinuity function \(f(x)=\left\{ \begin{align} & \frac{{{x}^{2}}-1}{x-1},\ \ x\ne 0 \\ & 3,\ \ \ \ \ \ \ \ x=0 \\\end{align} \right\}\).
Solution: The function is defined away from the point x = 1
In fact, if x ≠ 1, the function is
\(f(x)=\frac{{{x}^{2}}-1}{x-1}\),
\(=\frac{(x-1)(x+1)}{(x-1)}\),
= (x + 1)
However, if consider the point x = 1, this definition no longer makes sense since we would have to divide by zero. The function instead tells us that the value of the function is f (1) = 3.
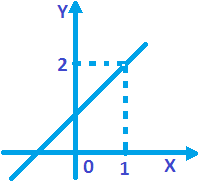
In this example, the graph has a hole at the point x = 1, which can be filled by redefined f(x) at x = 1 as 2 see in fig.
This type of discontinuity is called missing point discontinuity.
