Length of the Tangent from a Point to a Circle

Length of the Tangent from a Point to a Circle

Let the circle x²+ y² + 2gx + 2fy + c = 0. Then the center and the radius of the circleis are (-g, -f) and √ (g² +f² – c) respectively.

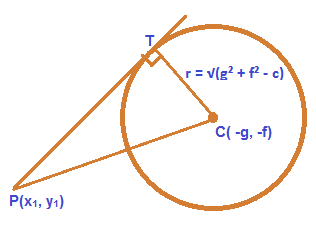
Let P (x₁, y₁) be any point outside the circle

In ΔPCT

\(PT=\sqrt{{{(PC)}^{2}}-{{(CT)}^{2}}}\).

\(PT=\sqrt{{{({{x}_{1}}+g)}^{2}}-{{({{y}_{1}}+f)}^{2}}-{{g}^{2}}-{{f}^{2}}+c}\).

\(PT=\sqrt{{{({{x}_{1}})}^{2}}+{{({{y}_{1}})}^{2}}+2g{{x}_{1}}+2f{{y}_{1}}+c}\).

PT = √S₁

Where S₁ = x₁² + y₁² + 2gx₁ + 2fy₁ + c = 0

Example: find the length of tangent from a point (1, 1) to a circle x² + y² + 4y + 2x = 4.

Solution: Given that,

x² + y² + 4y + 2x = 4, P (1, 1)

The centerand the radius of the circle is are (-1, -2)

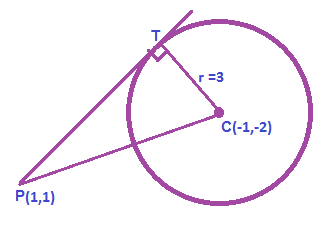
and √ (2² + 1² + 4) = 3, respectively.

S₁ = x₁² + y₁² + 4y₁ + 2x₁ = 4. At P (1, 1)

= 1 + 1 + 4 + 2 – 4

= 4

PT = √S₁

= √4

= 2.