When the mechanical properties of the system do not change then the system is in isolated state and it is called an isolated system. These systems are not interacting with environment and also called system with constant motion. The conservation laws are for isolated system because their properties are conserved and do not change. These laws are fundamental laws of mechanics in which the quantities like energy, angular momentum, and momentum etc. which are conserved quantities.
In the conservation law of energy, the energy is conserved and it is only converted from its one form to another form to make the total energy constant. Here, we discuss about the conservation of momentum in which the momentum of a system remains constant. Momentum is the mass in motion. It’s equal to mass multiplied by velocity. So, it depends on two factors that are how much and how fast an object is moved.
So, in the conservation law of momentum, the sum of all object momentum remains constant in interaction within the system. It is true for all types of momentum like angular, linear etc.
What Conservation of Momentum?
The law of conservation of momentum states that the momentum will remain constant no matter what until and unless any external force comes into action.
This results into the fact that the centre of mass of the system of objects will move with the same or constant velocity unless and until it is being acted upon by external force.
The Conservation of momentum is mathematically the result of the homogeneity of space, i.e., Conservation of momentum implies that the physical laws are independent of the position. Yet another consequence of the conservation of momentum is the Newton’s third law of motion that is the law of reciprocal actions. Let’s take an example when a gun is fired if we assume that the initial position was at rest and hence the initial momentum to be zero the final momentum should also be zero according to the law of conservation of momentum.
How to find the Rate of change of Momentum?
Newton’s Second law relates force with the rate of change of momentum. According to the law, force is directly proportional to the rate of change in momentum.
F α ΔP
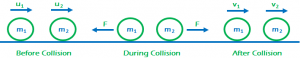
We will use this state law of conservation of momentum. According to this if the net force acting on the system is zero then the momentum of the system remains conserved. In other words, the change in momentum of the system is zero. We can see as F = 0, so will also be zero according to the second law. Let’s take the following example:-
We consider m₁ and m₂ as our system. So during the collision. The net force on the system is zero and hence we can conserve momentum of the system. The equation for momentum will be:
Initial Momentum = m₁u₁ + m₂u₂
Final Momentum = m₁v₁ + m₂v₂
So, according to conservation of momentum
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
But one thing to take care is that conservation is only true for system and not one body because if we consider only a single body or, then net force will be acting on it so we can’t write
m₁u₁ ≠ m₁v₁ (or) m₂u₂ ≠ m₂v₂.
Formula to find the Conservation of Momentum: For two objects with initial masses of m1 and m2 and initial velocity of u1 and u2 with final velocities after collision to be v1 and v2, we can write the law as,
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
Also, Δp₁ = – Δp₂
Let us consider an example of two objects such that they collide and apply equal and opposite force on each other according to the third law of Newton i.e., F₁ = – F₂
Also, it can be said that the time for which the force acted on object one is equal to the time for which the force acted on object two.
Hence, T₁ = T₂
So, the impulse experienced by both the object should also be same.
F₁T₁ = – F₂T₂
Also, the impulse is equal to the change in momentum.
M₁V₁ + M₂V₂ = 0, Momentum is conserved.
The vector sum of all the given momenta for a closed system with no external force acting on it remains constant:
P₁ + P₂ + P₃ + P₄ + … + Pᵢ = K, where K is a constant.
Examples of Conservation of Momentum:
- When atoms collide with each other the law of conservation of momentum is followed.
- When the bullet hits the wooden block the law of conservation of momentum is followed and the block rises to a particular height.
- When two cars hit each other in an accident then the law of conservation of momentum is followed.
- When a baseball is hit by the bat the law of conservation of momentum is followed.
- When two runners collide in a race then the law is followed.
- Two billiard balls colliding with each other.
How to find the Conservation of Momentum?
Example: A mass of 4 kg is moving at a speed of 10m/sec in a frictionless surface. It collides with a 3 kg mass moving in the same direction at 5 m/sec. what is the final velocity of the system after the collision?
Solution: Using Conservation of momentum:
m₁v₁ + m₂v₂ = (m₁ + m₂) vf
4 x 10 + 3 x 7 = 7 x vf
vf = 7.857 m/sec.
