When light travels from an optically denser medium to a rarer medium at the interface, it is partly reflected back in to the same medium and partly refracted to the second medium. This reflection is called the internal reflection.
When a ray of light enters from a denser medium to a rarer medium. It bends away from the normal, for example, the ray AO₁B in figure. The incident ray AO₁ is partially reflected (O₁C) and partially transmitted (O₁B) or reflected, the angle of refraction (r) being larger than the angle of incidence (i) As the angle of incidence increases, so does the angle of refraction, till for the ray AO₃. The angle of refraction is π/2. The refracted ray is bent so much away from the normal that it grazes the surface at the interface between the two media. This is shown by the ray AO₃D in figure. If the angle of incidence is increased still further (e.g., the ray AO₄), refraction is not possible, and the incident ray is totally reflected.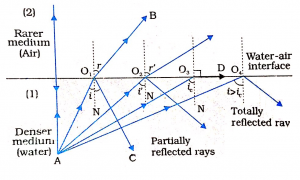 This is called total internal reflection, when light gets reflected by a surface, normally some fraction of it gets transmitted. The reflected ray, therefore, is always less intense than the incident ray, howsoever smooth the reflecting surface may be. In total internal reflection, on the other hand, no transmission of light takes place.
This is called total internal reflection, when light gets reflected by a surface, normally some fraction of it gets transmitted. The reflected ray, therefore, is always less intense than the incident ray, howsoever smooth the reflecting surface may be. In total internal reflection, on the other hand, no transmission of light takes place.
The angle of incidence corresponding to an angle of refraction 90⁰ say ∠AO₃N is called the critical angle (ic) for the given pair of media. We see from Snell’s law \(\left( \frac{\sin i}{\sin r}={{n}_{21}} \right)\) that if the relative refractive index is less than one then, since the maximum value of is unity, there is an upper limit to the value of sin i for which the law can be satisfied, that is, i = ic such that sin ic = n21.
