Evaluation of Trigonometric Limits

Evaluation of Trigonometric Limits

\(\underset{\theta \to 0}{\mathop{Lim}}\,\frac{\sin \theta }{\theta }=1\) (where θ is in radius)

Proof: Consider a circle of radius r. let O be the center of the circle such that \(\angle AOB=\theta \), where θ is measured in radians and its value is very small. Suppose the tangent at A meets OB produced at P. From fig we have

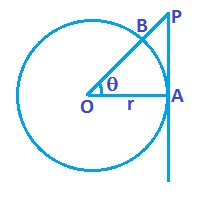
Area of ΔOAB < Area of sector OAB < Area of Δ OAP

\(\frac{1}{2}OA\times OB\sin \theta <\frac{1}{2}{{\left( OA \right)}^{2}}\theta <\frac{1}{2}OA\times OP\),

\(\frac{1}{2}{{r}^{2}}\sin \theta <\frac{1}{2}{{r}^{2}}\theta <\frac{1}{2}{{r}^{2}}\tan \theta \),

In triangle

AP = OA Tanθ

Sinθ < θ < tanθ

sinθ < θ < sinθ/cosθ

\(1<\frac{\theta }{\sin \theta }<\frac{1}{\cos \theta }\),

(since θ is small, sin θ > 0)

\(1>\underset{\theta \to 0}{\mathop{\lim }}\,\frac{\sin \theta }{\theta }>\underset{\theta \to 0}{\mathop{\lim }}\,\cos \theta \),

\(\underset{\theta \to 0}{\mathop{\lim }}\,\cos \theta <\underset{\theta \to 0}{\mathop{\lim }}\,\frac{\sin \theta }{\theta }<1\),

\(\cos 0<\underset{\theta \to 0}{\mathop{\lim }}\,\frac{\sin \theta }{\theta }<1\),

\(1<\underset{\theta \to 0}{\mathop{\lim }}\,\frac{\sin \theta }{\theta }<1\),

By sandwich theorem

\(\underset{\theta \to 0}{\mathop{\lim }}\,\frac{\sin \theta }{\theta }=1\),

Example: Evaluate the \(\underset{x\to 0}{\mathop{\lim }}\,\frac{\sin 3x}{x}\),

Solution:

We have  \(\underset{x\to 0}{\mathop{\lim }}\,\frac{\sin 3x}{x}\),

\(\underset{x\to 0}{\mathop{\lim }}\,\frac{3\sin 3x}{3x}\),

\(3\underset{x\to 0}{\mathop{\lim }}\,\frac{\sin 3x}{3x}\),

\(\left( \because \underset{x\to 0}{\mathop{\lim }}\,\frac{\sin 3x}{3x}=1 \right)\),

3(1) = 3