1. Vapour pressure of liquid-liquid solutions:
Raoult’s Law:- The partial pressure of each component in a binary volatile liquid-liquid solution at a given temperature is equal to the mole fraction of that component in the solution multiplied by the vapour pressure of that component in the pure state at same temperature.
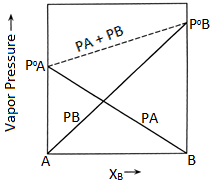
PA = [nA/ (nA + nB)] x P°A = XA * P°A
PB = ([nB/ (nA + nB)] x P°B = XB * P°B
Psolution = PA + PB = XA x P°A + XB x P°B
= (1 – XB) x P°A + XB x P°B
= P°A + (P°B – P°A) * XB
i.e., the total pressure varies linearly with mole fractions of the components
⇒ If component A is less volatile than component B i.e., P°A < P°B the minimum of Ptotal is P°A and maximum is P°B
⇒ If yA and yB are mole fractions of component A and B in vapour phase then according to Dalton’s law of partial pressures
PA = yA x Ptotal
PB = yB x Ptotal
Raoult’s law as a special case of Henry’s law:
⇒ According to Raoult’s law
PA = P°A * XA … (i)
⇒ According to Henry’s law for a gas in liquid solution the partial pressure of the volatile component, i.e., gas, is directly proportional to its mole fraction p α KH x … (ii)
⇒ In equation (i) and (ii), only the proportionality constant differs. Thus, Raoult’s law is a special case of Henry’s Law where KH = P°A
2. Vapour pressure of solid in liquid solution:
⇒ Non-volatile solute is added to a solvent then the vapour pressure is only due to the solvent. The vapour pressure of the solution decreases after adding solute.
⇒ The decrease in vapour pressure depends on the quantity of solute added (moles of solute).
According to Raoult’s law:
PA = P°A * XA
Where, A is the solvent.
P°A = vapour pressure of pure solvent.
The plot of PA and XA is a straight line.
