Trigonometric Ratios for Compound Angles – Part1
Cosine of the Difference of Two Angles:
\(\cos \left( A-B \right)=\cos A\cos B+\sin A\sin B\) for all angles A and B
Proof:
Let X’OX and YOY’ be the coordinate axes. Consider a unit circle with O as the center.
Let P₁, P₂ and P₃ be the three points on the circle such that \(\angle XO{{P}_{1}}=A,\,\,\angle XO{{P}_{2}}=B\ \ and\ \ \angle XO{{P}_{3}}=A-B\)
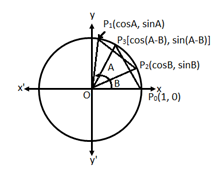
We know that equal chord of a circle makes equal angles at its center, so chord P₀P₃ and P₁P₂ subtend equal angles at O.
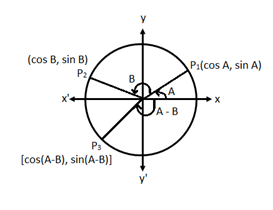
Therefore, \(Chord\ \ {{P}_{0}}{{P}_{3}}=\ Chord\ \ {{P}_{1}}{{P}_{2}}\),
\(\Rightarrow \sqrt{{{\left\{ \cos \left( A-B \right)-1 \right\}}^{2}}+{{\left\{ \sin \left( A-B \right)-0 \right\}}^{2}}}\)\(=\sqrt{{{\left\{ \cos B-\cos A \right\}}^{2}}+{{\left\{ \sin B-\sin A \right\}}^{2}}}\),
Squaring on both sides
\({{\cos }^{2}}\left( A-B \right)-2\cos \left( A-B \right)+1+{{\sin }^{2}}\left( A-B \right)\)\(={{\cos }^{2}}B+{{\cos }^{2}}A-2\cos A\cos B+{{\sin }^{2}}B+{{\sin }^{2}}A-2\sin A\sin B\),
\(\left( {{\cos }^{2}}\left( A-B \right)+{{\sin }^{2}}\left( A-B \right) \right)-2\cos \left( A-B \right)+1\)\(=\left( {{\cos }^{2}}B+{{\sin }^{2}}B \right)+\left( {{\cos }^{2}}A+{{\sin }^{2}}A \right)-2\cos A\cos B-2\sin A\sin B\),
\(\left( 1 \right)-2\cos \left( A-B \right)+1=\left( 1 \right)+\left( 1 \right)-2\cos A\cos B-2\sin A\sin B\),
\(2-2\cos \left( A-B \right)=2-2\cos A\cos B-2\sin A\sin B\),
\(1-\cos \left( A-B \right)=1-\cos A\cos B-\sin A\sin B\),
\(\cos \left( A-B \right)=\cos A\cos B+\sin A\sin B\).
