Speed of Wave Motion
A wave is a disturbance that moves along a medium from one end to the other. If one watches an ocean wave moving along the medium (the ocean water), one can observe that the crest of the wave is moving from one location to another over a given interval of time. The crest is observed to cover distance. The speed of an object refers to how fast an object is moving and is usually expressed as the distance travelled per time of travel. In the case of a wave, the speed is the distance travelled by a given point on the wave (such as a crest) in a given interval of time. To understand wave motion we have to know about the 3 basic properties of waves, let us visualize this better with the following diagram.
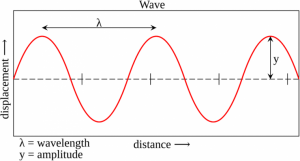
Amplitude: The amplitude y for the wave is given by the distance from the centre line to the tip of the crest or the tip of a trough, the amplitude of the wave tells about the energy of the wave, amplitude is measured in meters (m).
Wavelength: Wavelength is denoted by the symbol λ, it’s the Greek letter ‘Lambda’, well wavelength is the distance between two consecutive crest and trough. Wavelength is also measured in meters (m).
Frequency: It is denoted by f and is the number of waves passing through a point in one second; it is measured in hertz (Hz). Waves we find in day to day life like radio waves, microwaves have very high frequency so we make use of multiple of hertz like kilo-hertz, mega-hertz.
1 mega-hertz = 1000 kilo-hertz = 1000000 hertz.
Speed of a wave is how far a wave travels in one second, it is calculated in meters per second. The speed of light is around 3 x 108 m/s whereas the speed of sound is just 380m/s, because they are two different types of waves,
Waves are categorised based on the way they propagate:
- Transverse Waves: Waves in which the particles in their medium move at right angles to the direction of wave motion, example electromagnetic waves.
- Longitudinal Waves: Waves in which particles move in the direction of wave propagation, example sound waves. These waves travel in form of compression and rarefaction.
Speed of waves on string depends upon tension and mass density of the string and is given by V = √T/μ.
Wave speed on a stretched string depends on the tension in the string and the linear density of the string and not on the frequency of the wave, so changing the mass density or the tension in the string will affect the velocity of the wave.
