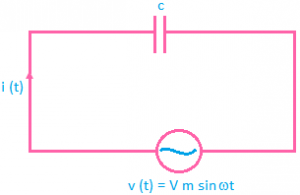
 \(i\left( t \right)=C\frac{dV}{dt}=C\frac{d}{dt}~\left( {{V}_{m}}~sin\omega t \right)\).
\(i\left( t \right)=C\frac{dV}{dt}=C\frac{d}{dt}~\left( {{V}_{m}}~sin\omega t \right)\).
\(=\omega C{{V}_{m}}\cos \omega t=~\frac{~{{V}_{m}}}{\frac{1}{\omega C}}\text{sin}\left( \omega t+{{90}^{0}} \right)\).
\(=\frac{{{V}_{m}}}{{{X}_{c}}}\sin \left( \omega t+{{90}^{0}} \right)\).
Where, \({{X}_{C}}=\frac{1}{\omega C}\) and is known as capacitive reactance
i (t) = Im sin (ωt + 90⁰)
Instantaneous power
p (t) = v(t) i(t)
= Vm sinωt Im cos ωt
\(P\left( t \right)=\frac{{{V}_{m}}{{I}_{m}}}{2}\sin 2\omega t\).
Average power
\({{P}_{avg}}=\frac{1}{2\pi }~\underset{0}{\overset{2\pi }{\mathop \int }}\,p\left( t \right)~d\omega t=0~\).
Now vector diagrams are drawn below: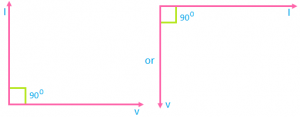 Hence in a capacitor current leads to voltage by 90° (or voltage lags to currents by 90°).
Hence in a capacitor current leads to voltage by 90° (or voltage lags to currents by 90°).
