Here goes the revision tips for a quick recollection of the important content in the chapter COMPLEX NUMBERS.
Equation of perpendicular bisector: The equation of the perpendicular bisector of the line segment joining points z₁ and z₂ is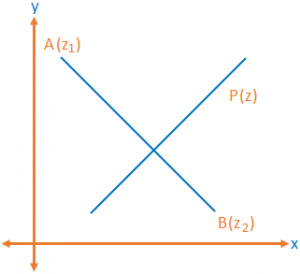
z (z₁̅ – z₂̅) + z̅ (z₁ – z₂) = |z₁|² – |z₂|².
Important results: If z₁, z₂, z₃ are the points A, B and C in argand plane then
\(\angle BAC=\arg \left( \frac{{{z}_{3}}-{{z}_{1}}}{{{z}_{2}}-{{z}_{1}}} \right)\).
\(\frac{{{z}_{3}}-{{z}_{1}}}{{{z}_{2}}-{{z}_{1}}}=\left| \frac{{{z}_{3}}-{{z}_{1}}}{{{z}_{2}}-{{z}_{1}}} \right|(\cos \alpha +i\sin \alpha ),\alpha =\angle BAC\)Two triangles z₁, z₂, z₃ are vertices of ABC and a₁, a₂, a₃ vertices of DEF are similar if \(\left| \begin{matrix}{{z}_{1}} & {{a}_{1}} & 1\\{{z}_{2}} & {{a}_{2}} & 1 \\{{z}_{3}} & {{a}_{3}} & 1 \\\end{matrix} \right|=0\).
The equation of circle having z₁ and z₂ as the end points of the diameter is (z – z₁) (z̅ – z̅₂) + (z̅ – z̅₁) (z – z₂)
If z₁ and z₂ are fixed complex numbers then locus of a point Z satisfying \(\arg \left( \frac{z-{{z}_{1}}}{z-{{z}_{2}}} \right)=\pm \frac{\pi }{2}\) is a circle having z₁, z₂ as end points of diameter.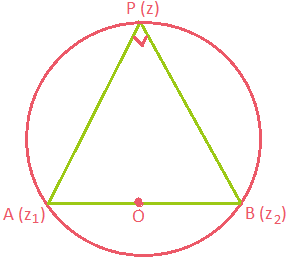
(i) The equation of circle passing through 3 points z₁, z₂, z₃ is \(\left( \frac{z-{{z}_{3}}}{z-{{z}_{1}}} \right)\left( \frac{{{z}_{2}}-{{z}_{1}}}{{{z}_{2}}-{{z}_{3}}} \right)=\left( \frac{\overline{z}-\overline{{{z}_{3}}}}{\overline{z}-\overline{{{z}_{1}}}} \right)\left( \frac{\overline{{{z}_{2}}}-\overline{{{z}_{1}}}}{\overline{{{z}_{2}}}-\overline{{{z}_{3}}}} \right)\).
(ii) If four points z₁, z₂, z₃ z₄ are concyclic \(\left( \frac{{{z}_{1}}-{{z}_{2}}}{{{z}_{1}}-{{z}_{4}}} \right)\left( \frac{{{z}_{3}}-{{z}_{4}}}{{{z}_{3}}-{{z}_{2}}} \right)\) must be purely real.
Some standard loci in the argand plane: If z is a variable point and z₁, z₂ are two fixed points in the argand plane then
(i) |z – z₁| = |z – z₂|
Locus of z is perpendicular bisector of line segment joining z₁ and z₂.
(ii) |z – z₁| + |z – z₂|=|z₁ – z₂|
Locus of z is line segment joining z₁ and z₂.
(iii) |z – z₁| + |z – z₂|= k (≠|z₁ – z₂|) locus of z is ellipse.
(iv) |z – z₁| – |z – z₂|= k |z₁ – z₂|.
Locus of z is a straight line joining z₁ and z₂ but does not lie between z₁ and z₂.
(v) |z – z₁| – |z – z₂|= k (≠|z₁ – z₂|).
Locus of z is hyperbola.
(vi) |z – z₁|² + |z – z₂|²= |z₁ – z₂|².
Locus of z is a circle with z₁ and z₂ as extremities of diameter.
(vii) |z – z₁| = k |z – z₂| k ≠ 1.
Locus of z is a circle.
(viii) \(\arg \left( \frac{z-{{z}_{1}}}{z-{{z}_{2}}} \right)=\alpha \,(fixed)\).
Locus of z is segment of circle
(ix) \(\arg \left( \frac{z-{{z}_{1}}}{z-{{z}_{2}}} \right)=0\,(or)\pi \).
Then locus of z is straight line through z₁, z₂.
If z₁, z₂, z₃ are vertices of a triangle ABC then its orthocenter is \(=\frac{(a\sec A){{z}_{1}}+(b\sec B){{z}_{2}}+(c\sec C){{z}_{3}}}{a\sec A+b\sec B+c\sec C}\).
The circumcenter of with z₁, z₂, z₃ as vertices of triangle is \(z=\frac{\sum{{{z}_{1}}\overline{{{z}_{1}}}({{z}_{2}}-{{z}_{3}})}}{\sum{\overline{{{z}_{1}}}({{z}_{2}}-{{z}_{3}})}}\).
Orthocenter will be \(z=\frac{\sum{{{z}^{2}}_{1}(\overline{{{z}_{2}}}-\overline{{{z}_{3}}})}+\sum{{{z}_{1}}^{2}({{z}_{2}}-{{z}_{3}})}}{\sum{({{z}_{1}}\overline{{{z}_{2}}}-\overline{{{z}_{1}}}{{z}_{2}})}}\).
If z₁, z₂, z₃ are vertices of a triangle, if the triangle is equilateral then \(\frac{1}{{{z}_{2}}-{{z}_{3}}}+\frac{1}{{{z}_{3}}-{{z}_{1}}}+\frac{1}{{{z}_{1}}-{{z}_{2}}}=0\).
\({{z}^{2}}_{1}+{{z}_{2}}^{2}+{{z}_{3}}^{3}={{z}_{1}}{{z}_{2}}+{{z}_{2}}{{z}_{3}}+{{z}_{3}}{{z}_{1}}\).
Let zₒ be circumcentre then \({{z}^{2}}_{1}+{{z}_{2}}^{2}+{{z}_{3}}^{3}=3{{z}^{2}}_{0}\).