Properties of Triangle – Theorem
m – n Theorem:
Let D be a point on the side BC of Triangle ABC such that BD. DC = m: n and angle BAD = α, angle ADC = θ and angle DAC = β then (m + n) cot θ = m cotα – n cotβ
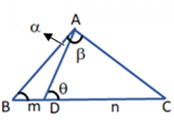
Proof:
BD/DC = m/n
And angle ADC = θ
\(\angle ADB=\left({{180}^{o}}-\theta \right)\),
\(\angle BAD=\alpha \ \ and\ \ \angle DAC=\beta \),
\(\angle ABD={{180}^{o}}-\left( \alpha+{{180}^{o}}-\theta \right)=\theta -\alpha =\beta \),
From ΔABD,
BD/sinα = AD/sin(θ – α)…(i)
From ΔADC,
DC/sinβ= AD/sin(θ + β)…(ii)
From equation i/ii
\(\frac{BD/sin\alpha }{DC/sin\beta }=\frac{AD/sin\left( \theta \text{ }\text{ }\alpha \right)}{AD/sin\left( \theta \text{ }+\text{ }\beta \right)}\),
\(\frac{BD/sin\beta }{DC/sin\alpha}=\frac{\sin \left( \theta +\beta \right)}{\sin \left( \theta -\alpha \right)}\),
(since using sin(x+y) and sin(x-y) formulas)
\(\frac{BDsin\beta }{DCsin\alpha }=\frac{\sin \theta \cos \beta +\cos \theta \sin \beta }{\sin \theta \cos \alpha -\cos \theta \sin \alpha }\),
BD/DC = m/n
\(\frac{m\ sin\beta }{n\ sin\alpha }=\frac{\sin \theta \cos \beta +\cos \theta \sin \beta }{\sin \theta \cos \alpha -\cos \theta \sin \alpha }\),
m sin β(sin θ cos α -cos θ sin α) = n sin α (sinθ cos β + cosθ sin β )
dividing both sides by sinα sinβ sinθ
m cot α – m cot θ = n cot β + n cot θ
m cot α – n cot β = n cot θ + m cot θ
cot θ (m+ n) = m cot α – n cot β
Example: If the median AD of triangle ABC makes an angle π/4 with side BC, then find the valve of
|cotB – cotC|.
solution:
by m- n theorem
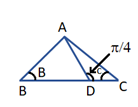
cot θ (m+ n) = m cot α – n cot β
(BD+DC) cot π/4 = DC cotB – BD cotC
|cotB – cotC| = 2
