Perpendicular Distance of a Point from a Line – Vector Form

Perpendicular Distance of a Point from a Line – Vector Form

Vector Form:

Let L be the foot of the perpendicular draw from \(P(\overrightarrow{\alpha })\) on the line \(\vec{r}=\vec{a}+\lambda \vec{b}\).

Since \(\vec{r}\) denotes the position vector of any point on the line \(\vec{r}=\vec{a}+\lambda \vec{b}\), the position vector of L will be \((\vec{a}+\lambda \vec{b})\).

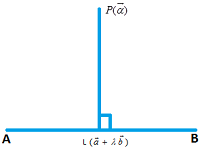
Direction ratios of PL = \(\vec{a}-\vec{\alpha }+\lambda \vec{b}\).

Since \(\overrightarrow{PL}\) is perpendicular to \(\overrightarrow{b}\), we have

\(\left( \vec{a}-\vec{\alpha }+\lambda \vec{b} \right).\vec{b}=0\).

\(\left( \vec{a}-\vec{\alpha } \right).\vec{b}+\lambda \vec{b}.\vec{b}=0\).

\(\lambda =\frac{-\left( \vec{a}-\vec{\alpha } \right).\vec{b}}{{{\left| {\vec{b}} \right|}^{2}}}\).

Thus, position vector of L is  \(\vec{a}-\left( \frac{\left( \vec{a}-\vec{\alpha } \right).\vec{b}}{{{\left| {\vec{b}} \right|}^{2}}} \right)\vec{b}\), which is the foot of the perpendicular

Example: Find the coordinates of the foot of the perpendicular drawn from point A (1, 0, 3) to the join of points B (4, 7, 1) and C (3, 5, 3).

Solution: Point A (1, 0, 3) to the join of points B (4, 7, 1) and C (3, 5, 3).

Let D be the foot of the perpendicular and let it divide BC in the ratio . Then the coordinates of D are \(\frac{3\lambda +4}{\lambda +1},\frac{5\lambda +7}{\lambda +1},\frac{3\lambda +1}{\lambda +1}\).

Now, \(\overrightarrow{AD}\bot \overrightarrow{BC}\Rightarrow \overrightarrow{AD}.\overrightarrow{BC}=0\).

(2λ + 3) + 2 (5λ + 7) + 4 = 0.

\(\lambda =\frac{-7}{4}\).

\(\Rightarrow \frac{3\lambda +4}{\lambda +1}\).

\(\frac{3\left( \frac{-7}{4} \right)+4}{\left( \frac{-7}{4} \right)+1}=\frac{5}{3}\).

\(\Rightarrow \frac{5\lambda +7}{\lambda +1}\).

\(\lambda =\frac{-7}{4}\).

\(\frac{5\left( \frac{-7}{4} \right)+7}{\left( \frac{-7}{4} \right)+1}=\frac{7}{3}\).

\(\Rightarrow \frac{3\lambda +1}{\lambda +1}\).

\(\lambda =\frac{-7}{4}\).

\(\frac{3\left( \frac{-7}{4} \right)+1}{\left( \frac{-7}{4} \right)+1}=\frac{17}{3}\).

Thus, coordinates of D are 5/3, 7/3 and 17/3.