Parabola – Two parabolas intersect at an angle

Parabola – Two parabolas intersect at an angle

Prove that the two parabolas y² = 4ax and x² = 4by intersect (other then the origin) at an angle of \({{\operatorname{Tan}}^{-1}}\left[ \frac{3{{a}^{\frac{1}{3}}}{{b}^{\frac{1}{3}}}}{2\left( {{a}^{\frac{2}{3}}}+{{b}^{\frac{2}{3}}} \right)} \right]\).

Solution: Without loss of generality we can assume that a > 0 and b > 0

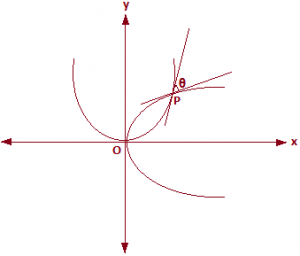
Let P (x, y) be the point of intersection of the parabolas other than the origin then y⁴ = 16a²x²

16 a² (4by) = 64 a²by

∴ y [y³ – 64a²b] = 0

y³ – 64a²b = 0

y = (64a²b)^⅓ = 4a^⅔b^⅓

From y² = 4ax, we get  \(x=\frac{16{{a}^{4/3}}{{b}^{2/3}}}{4a}=4{{a}^{1/3}}{{b}^{2/3}}\),

∴ P = (4a^⅓b^⅔, 4a^⅔b^⅓)

Differentiating both sides of y² = 4ax, w.r.t ‘x’ we get

\(\frac{dy}{dx}=\frac{2a}{y}\),

∴ \(\left[ \frac{dy}{dx} \right]=\frac{2a}{4{{a}^{2/3}}{{b}^{1/3}}}=\frac{1}{2}{{\left( \frac{a}{b} \right)}^{1/3}}\),

If m₂ is the slope of tangent at P to y² = 4ax then we get  \({{m}_{1}}=\frac{1}{2}{{\left( \frac{a}{b} \right)}^{1/3}}\),

Similarly, if m₁ is the slope of the tangent at P to x² = 4 by then we get \({{m}_{2}}=2{{\left( \frac{a}{b} \right)}^{1/3}}\),

If the θ is the acute angle between the tangent to the curves at P, then \(Tan\theta =\left| \frac{{{m}_{2}}-{{m}_{1}}}{1+{{m}_{1}}{{m}_{2}}} \right|\),

\(Tan\theta =\frac{3{{a}^{\frac{1}{3}}}{{b}^{\frac{1}{3}}}}{2\left( {{a}^{\frac{2}{3}}}+{{b}^{\frac{2}{3}}} \right)}\),

\(\theta ={{\operatorname{Tan}}^{-1}}\left[ \frac{3{{a}^{\frac{1}{3}}}{{b}^{\frac{1}{3}}}}{2\left( {{a}^{\frac{2}{3}}}+{{b}^{\frac{2}{3}}} \right)} \right]\).