Pair of straight lines – Theorem

Pair of straight lines – Theorem

Statement: The product of the perpendicular from (α, β) to the pair of lines ax² + 2hxy + by² = 0 is \(\frac{\left| a{{\alpha }^{2}}+2h\alpha \beta +b{{\beta }^{2}} \right|}{\sqrt{{{(a-b)}^{2}}+4{{h}^{2}}}}\).

Proof: Let ax² + 2hxy + by² = 0 … (1)

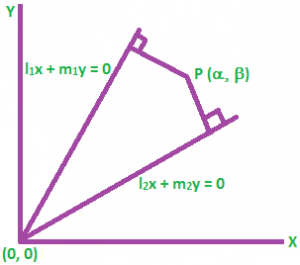
Represent lines l₁ x + m₁ y = 0 and l₂ x + m₂ y = 0

L₁L₂ = 0 represent a pair of straight line

(l₁ x + m₁ y) (l₂ x + m₂ y) = 0

l₁ l₂x² + m₁ m₂ y² + (l₁ m₂ + l₂ m₁) x y = 0 … (2)

compare equation 1 and 2 we get a, b and 2h

l₁ l₂ = a,

m₁ m₂ = b

 and l₁ m₂ + l₂ m₁ = 2h

length of the perpendicular from (α, β) to l₁ x + m₁ y = 0

\(P=\frac{\left| {{l}_{1}}\alpha +{{m}_{1}}\beta  \right|}{\sqrt{{{l}_{1}}^{2}+{{m}_{1}}^{2}}}\).

Length of the perpendicular from (α, β) to l₂ x + m₂ y = 0

\(q=\frac{\left| {{l}_{2}}\alpha +{{m}_{2}}\beta  \right|}{\sqrt{{{l}_{2}}^{2}+{{m}_{2}}^{2}}}\).

The product of the perpendicular from (α, β) to the pair of lines ax² + 2hxy + by² = 0 is

\(pq=\frac{\left| {{l}_{1}}\alpha +{{m}_{1}}\beta  \right|}{\sqrt{{{l}_{1}}^{2}+{{m}_{1}}^{2}}}.\frac{\left| {{l}_{2}}\alpha +{{m}_{2}}\beta  \right|}{\sqrt{{{l}_{2}}^{2}+{{m}_{2}}^{2}}}\),

\(pq=\frac{\left| {{l}_{1}}{{l}_{2}}{{\alpha }^{2}}+{{m}_{1}}{{m}_{2}}{{\beta }^{2}}+({{l}_{1}}{{m}_{2}}+{{l}_{2}}{{m}_{1}})\alpha \beta  \right|}{\sqrt{({{l}_{2}}^{2}+{{m}_{2}}^{2})({{l}_{1}}^{2}+{{m}_{1}}^{2})}}\),

\(pq=\frac{\left| {{l}_{1}}{{l}_{2}}{{\alpha }^{2}}+{{m}_{1}}{{m}_{2}}{{\beta }^{2}}+({{l}_{1}}{{m}_{2}}+{{l}_{2}}{{m}_{1}})\alpha \beta  \right|}{\sqrt{{{l}_{1}}^{2}{{l}_{2}}^{2}+{{l}_{1}}^{2}{{m}_{2}}^{2}+{{m}_{1}}^{2}{{l}_{2}}^{2}+{{m}_{1}}^{2}{{m}_{2}}^{2}}}\),

\(pq=\frac{\left| {{l}_{1}}{{l}_{2}}{{\alpha }^{2}}+{{m}_{1}}{{m}_{2}}{{\beta }^{2}}+({{l}_{1}}{{m}_{2}}+{{l}_{2}}{{m}_{1}})\alpha \beta  \right|}{\sqrt{{{({{l}_{1}}{{l}_{2}}+{{m}_{1}}{{m}_{2}})}^{2}}-2{{l}_{1}}{{l}_{2}}{{m}_{1}}{{m}_{2}}+{{({{l}_{1}}{{m}_{2}}+{{l}_{2}}{{m}_{1}})}^{2}}+2{{l}_{1}}{{l}_{2}}{{m}_{1}}{{m}_{2}}}}\),

\(pq=\frac{\left| {{l}_{1}}{{l}_{2}}{{\alpha }^{2}}+{{m}_{1}}{{m}_{2}}{{\beta }^{2}}+({{l}_{1}}{{m}_{2}}+{{l}_{2}}{{m}_{1}})\alpha \beta  \right|}{\sqrt{{{({{l}_{1}}{{l}_{2}}+{{m}_{1}}{{m}_{2}})}^{2}}+{{({{l}_{1}}{{m}_{2}}+{{l}_{2}}{{m}_{1}})}^{2}}}}\).

We know that l₁ l₂ = a, m₁ m₂ = b, l₁ m₂ + l₂ m₁ = 2h these values are substitute above equation.

\(pq=\frac{\left| a{{\alpha }^{2}}+2h\alpha \beta +b{{\beta }^{2}} \right|}{\sqrt{{{(a+b)}^{2}}+{{(2h)}^{2}}}}\),

\(pq=\frac{\left| a{{\alpha }^{2}}+2h\alpha \beta +b{{\beta }^{2}} \right|}{\sqrt{{{(a+b)}^{2}}+4{{h}^{2}}}}\).

Hence proved the product of the perpendicular from (α, β) to the pair of lines ax² + 2hxy + by² = 0 is \(\frac{\left| a{{\alpha }^{2}}+2h\alpha \beta +b{{\beta }^{2}} \right|}{\sqrt{{{(a-b)}^{2}}+4{{h}^{2}}}}\).

Example: The product of the perpendicular from (1, 2) to the pair of lines 2x² – xy + y² = 0 is

Solution: Given that

2x² – xy + y² = 0 and (1, 2)

Where a = 2, b = 1 and h= -½

We know that the product of the perpendicular from (α, β) to the pair of lines ax² + 2hxy + by² = 0 is \(\frac{\left| a{{\alpha }^{2}}+2h\alpha \beta +b{{\beta }^{2}} \right|}{\sqrt{{{(a-b)}^{2}}+4{{h}^{2}}}}\),

= \(\frac{\left| 2{{x}^{2}}-xy+{{y}^{2}} \right|}{\sqrt{{{(2-1)}^{2}}+4{{\left( \frac{-1}{2} \right)}^{2}}}}\) ,

= \(\frac{\left| 2{{(1)}^{2}}-(1)(2)+{{(2)}^{2}} \right|}{\sqrt{{{(2-1)}^{2}}+4\left( \frac{1}{4} \right)}}\),

= \(\frac{\left| 2-2+4 \right|}{\sqrt{1+1}}\),

 = 2√2.