Length of the Latus Rectum and Parametric Equation of Chord

Length of the Latus Rectum and Parametric Equation of Chord

Length of the Latus Rectum: The length of the latus rectum of the parabola y² = 4ax is 4a

Proof: Let LL’ be the length of the latus rectum of the parabola y² = 4ax

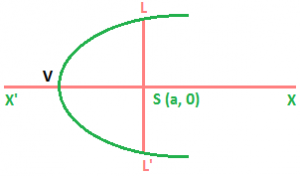
Let SL = I, then L = (a, l)

Since L is a point on the parabola y² = 4ax

x = a, y = l substitute above equation

l² = 4a(a)

l² = 4a²

l = 2a

SL = 2a

∴ LL’ = 2SL = 4a

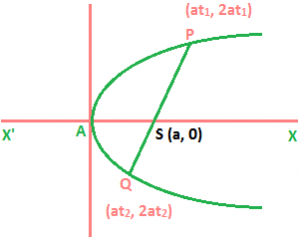
Parametric Equation of Chord: Let P (at₁², 2at₁) and Q (at₂², 2at₂) be any point on the parabola y² = 4ax then equation of chord PQ is

PQ Slope(m) = \(\frac{2a{{t}_{2}}-2a{{t}_{1}}}{a{{t}_{2}}^{2}-a{{t}_{1}}^{2}}\),

\(\left( y\text{ }-\text{ }2at \right)\text{ }=\frac{2a{{t}_{2}}-2a{{t}_{1}}}{a{{t}_{2}}^{2}-a{{t}_{1}}^{2}}(x-a{{t}_{1}}^{2})\) [∵ (y – y₁) = m (x – x₁)],

\((y-2a{{t}_{1}})=\frac{2a{{t}_{2}}-2a{{t}_{1}}}{a({{t}_{2}}-{{t}_{1}})({{t}_{2}}+{{t}_{1}})}(x-a{{t}_{1}}^{2})\),

\((y-2a{{t}_{1}})=\frac{2a({{t}_{2}}-{{t}_{1}})}{a({{t}_{2}}-{{t}_{1}})({{t}_{2}}+{{t}_{1}})}(x-a{{t}_{1}}^{2})\),

\(\left( y\text{ }-\text{ }2at \right)\text{ }=\frac{2}{{{t}_{1}}+{{t}_{2}}}(x-a{{t}_{1}}^{2})\).

y (t₁ + t₂) = 2x + 2at₁t₂

∴ Equation of chord joining points t₁ and t₂ on the parabola is y (t₁ + t₂) = 2x + 2at₁t₂.

If the chord PQ is focal chord then it passes through (a,0)

y (t₁ + t₂) = 2x + 2at₁t₂

x = a, y = 0

0 (t₁ + t₂) = 2(a) + 2at₁t₂

2a + 2at₁t₂ = 0

t₁t₂ = -1

For focal chord PQ of parabola

y² = 4ax if P is (at², 2at)

Q is \(\left( \frac{a}{{{t}^{2}}},\frac{-2a}{t} \right)\).