Dear all, you might all be afraid of integration though it is quite fascinating topic… Well, here we bring up few important concepts in integration, by which you can make your preparation interesting…!!
Indefinite Integrals:
A function ∅ (x) is called a primitive (or) an anti-derivative of a function f(x) of [∅’ (x) = f (x)]
⇒ ∫f(x) = ∅ (x) + C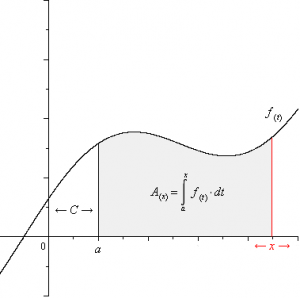 Here f(x) = integrand
Here f(x) = integrand
\(\frac{d\left( \int{f(x)dx} \right)}{dx}=f(x)\).
Algorithm to solve integrals of form:
∫sinmx cosnx dx, ∫sinmx dx and ∫cosnx dx where [m, n ϵ N]
Step 1: Find m, n
Step 2: If m is odd i.e. power or index of sin x is odd, put cos x = t and reduced the integrand in terms of it
If n is odd then put sin x = t and reduce the integrand
If both are odd you can use any of above two methods
To evaluate integrals of form ∫sinmx cosnx dx where [m, n ϵ Q] and m + n is negative even integer then put tan x = t.
To evaluate integrals of form ∫sinmx cosnx dx where m, n are positive even integers then.
Let Z = cos x + i sin x
1/Z = cosx – i sinx
\(Z+\frac{1}{Z}=2\cos x\).
\(Z-\frac{1}{Z}=2i\sin x\).
\(\cos nx=\frac{1}{2}\left( {{Z}^{n}}+\frac{1}{{{Z}^{n}}} \right)\).
\(\sin nx=\frac{1}{2i}\left( {{Z}^{n}}-\frac{1}{{{Z}^{n}}} \right)\).
Some important standard integrals:
\(\int{\frac{1}{{{x}^{2}}-{{a}^{2}}}dx=\frac{1}{2a}\log \left| \frac{x-a}{x+a} \right|+C}\).
\(\int{\frac{1}{{{a}^{2}}-{{x}^{2}}}dx=\frac{1}{2a}\log \left| \frac{a+x}{a-x} \right|+C}\).
\(\int{\frac{1}{\sqrt{{{a}^{2}}+{{x}^{2}}}}dx=\log \left| x+\sqrt{{{a}^{2}}+{{x}^{2}}} \right|+C}\).
\(\int{\frac{1}{\sqrt{{{x}^{2}}-{{a}^{2}}}}dx=\log \left| x+\sqrt{{{x}^{2}}-{{a}^{2}}} \right|+C}\).
Integrals of form
\(\int{\frac{dx}{{{\left( a+b\cos x \right)}^{2}}}},\,\int{\frac{dx}{{{\left( a+b\sin x \right)}^{2}}}}\) to evaluate this type of integrals
Define \(P=\frac{\sin x}{a+b\cos x}\,\left( or \right)\,\frac{\cos x}{a+b\sin x}\).
Depending on integral then find \(\frac{dP}{dx}\) interms of \(\frac{1}{a+b\cos x}\,\left( or \right)\,\frac{1}{a+b\sin x}\).
Now integrate both sides of expression to get required integral.
Integration by parts:
\(\int{uvdx=u\int{vdx-\int{\left\{ \frac{du}{dx}\int{vdx} \right\}}dx}}\).
Very IMP Note:
Choose first function as the function which come first in word “ILATE”
I – Inverse trigonometric
L – Logarithmic functions
A – Algebraic functions
T for Trigonometric functions
E for exponential functions
Integrals of the form ∫ex [f(x) + f’(x)] dx = ex f(x) + C
Note:
∫ekx [k f(x) + f’(x)] = ekx f(x) + C
\(\int{{{e}^{ax}}\sin bx=\frac{{{e}^{ax}}}{{{a}^{2}}+{{b}^{2}}}\left( a\sin bx+b\cos bx \right)}+C\).
\(\int{{{e}^{ax}}\cos bx=\frac{{{e}^{ax}}}{{{a}^{2}}+{{b}^{2}}}\left( a\cos bx+b\sin bx \right)}+C\).
Some very important integrals:
i) \(\int{\sqrt{{{a}^{2}}-{{x}^{2}}}dx=\frac{x}{2}\sqrt{{{a}^{2}}-{{x}^{2}}}+\frac{1}{2}{{a}^{2}}{{\sin }^{-1}}\left( \frac{x}{a} \right)+C}\).
ii) \(\int{\sqrt{{{a}^{2}}+{{x}^{2}}}dx=\frac{x}{2}\sqrt{{{a}^{2}}+{{x}^{2}}}+\frac{{{a}^{2}}}{2}\log \left| x+\sqrt{{{a}^{2}}+{{x}^{2}}} \right|+C}\).
iii) \(\int{\sqrt{{{x}^{2}}-{{a}^{2}}}dx=\frac{x}{2}\sqrt{{{x}^{2}}-{{a}^{2}}}-\frac{{{a}^{2}}}{2}\log \left| x+\sqrt{{{x}^{2}}-{{a}^{2}}} \right|+C}\).
Reduction formulae:
1) ∫sinn x dx = In
\({{I}_{n}}=\frac{-{{\sin }^{n-1}}x\cos x}{n}+\frac{n-1}{n}{{I}_{n-2}}\).
2) ∫cosnx dx = In
\({{I}_{n}}=\frac{-{{\cos }^{n-1}}x\sin x}{n}+\frac{n-1}{n}{{I}_{n-2}}\).
3) ∫tann x dx = In
\({{I}_{n}}=\frac{{{\tan }^{n-1}}x}{n-1}-{{I}_{n-2}}\).
4) ∫cosecn x dx = In
\({{I}_{n}}=\frac{-\cos e{{c}^{n-2}}x\cot x}{n-1}+\frac{n-2}{n-1}{{I}_{n-2}}\).
5) ∫secn x dx = In
\({{I}_{n}}=\frac{-se{{c}^{n-2}}x\tan x}{n-1}+\frac{n-2}{n-1}{{I}_{n-2}}\).
6) ∫cotn x dx = In
\({{I}_{n}}=\frac{-{{\cot }^{n-1}}x}{n-1}-{{I}_{n-2}}\).
Your comments and questions are warmly welcomed….
