| Fundamental Terms | Hyperbola | Conjugate Hyperbola | |
| (a) | Equation | \(\frac{{{x}^{2}}}{{{a}^{2}}}-\frac{{{y}^{2}}}{{{b}^{2}}}=1\) |
\(-\frac{{{x}^{2}}}{{{a}^{2}}}+\frac{{{y}^{2}}}{{{b}^{2}}}=1\) |
|
(b) |
Graph | 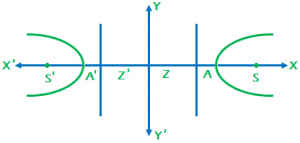 |
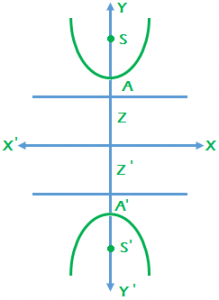 |
| (c) | Centre | C (0, 0) |
C (0, 0) |
|
(d) |
Vertices | (±a, 0) | (0, ±b) |
| (e) | Length of transverse axis | 2a |
2b |
|
(f) |
Length of conjugate axis | 2b | 2a |
| (g) | Foci | (±ae, 0) |
(0, ±be) |
|
(h) |
Equation of directrices | \(x=\pm \left( \frac{a}{e} \right)\) | \(y=\pm \left( \frac{b}{e} \right)\) |
| (i) | Eccentricity | \(e=\sqrt{1+\frac{{{b}^{2}}}{{{a}^{2}}}}\) |
\(e=\sqrt{1+\frac{{{a}^{2}}}{{{b}^{2}}}}\) |
|
(j) |
Length of latus rectum | \(\frac{2{{b}^{2}}}{a}\) | \(\frac{2{{a}^{2}}}{b}\) |
| (k) | Ends of latus rectum | \(\left( \pm ae,\pm \frac{{{b}^{2}}}{a} \right)\) |
\(\left( \pm \frac{{{a}^{2}}}{b},\pm be \right)\) |
|
(l) |
Parametric equations | \(\left\{ \begin{align}& x=a\sec \alpha \\& y=b\tan \alpha \\\end{align} \right\}\) or \(x=a\left( \frac{{{e}^{\theta }}+{{e}^{-\theta }}}{2} \right)\) | \(\left\{ \begin{align}& x=a\tan \alpha \\& y=b\sec \alpha \\\end{align} \right\}\) or \(y=\frac{{{e}^{\theta }}-{{e}^{-\theta }}}{2}\) |
| (m) | Parametric coordinates | (a secα, b tanα) |
(a tanα, b secα) |
|
(n) |
Foci radii | |SP| = (ex₁ – a) and |S¹P| = (ex₁ + a) | |SP| = (ey₁ – b) and |S¹P| = (ey₁ + b) |
| (o) | Difference of focal radii=|SP|-|S’P| | 2a |
2b |
|
(p) |
Distance between foci | 2ae | 2be |
| (q) | Tangents at vertices | x = a and x = – a |
y = b and y = -b |
