Geometrical Interpretation of definite Integral
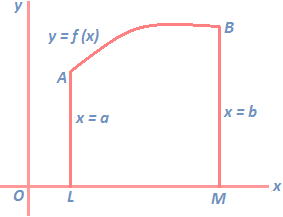
If f(x) > 0 for all x ϵ [a, b] then \(\int_{a}^{b}{f\left( x \right)}dx\) is numerical equal to the area bounded by the curve y = f(x) then x-axis and the straight lines x = a and x = b.
\(\int_{a}^{b}{f\left( x \right)}\left( dx \right)\) = Area LAMB.
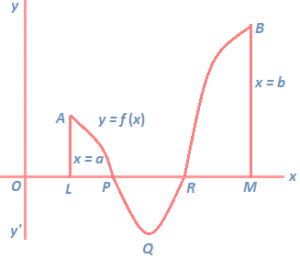
In general, \(\int_{a}^{b}{f\left( x \right)}dx\) represented an algebraic sum of the areas of the figure bounded by the curve y=f(x), the x-axis and the straight lines x = a and x = b. the areas above x-axis are taken with plus sign and the below x-axis are taken with a minus sign.
\(\int_{a}^{b}{f\left( x \right)dx}\) = Area LAP – Area PQR + Area RBM.
Evaluate: \(\int_{a}^{b}{{{e}^{x}}}dx\) a limit of suns.
Solution: \(\underset{h\to 0}{\mathop{\lim }}\,h\left[ f\left( a \right)+f\left( a+h \right)+f\left( a+2h \right)+….+f\left( a+\left( n-1 \right)h \right) \right]\) where \(h=\frac{b-a}{n}\).
Here, f(x) = ex
∴ \(\int_{a}^{b}{{{e}^{x}}}dx\),
\(=\underset{h\to 0}{\mathop{\lim }}\,h\left[ {{e}^{a}}+{{e}^{a+h}}+{{e}^{a+2h}}+….+{{e}^{a+\left( n-1 \right)h}} \right]\),
\(=\underset{h\to 0}{\mathop{\lim }}\,h{{e}^{a}}\left[ 1+{{e}^{h}}+{{2}^{2h}}+{{e}^{3h}}+….+{{e}^{\left( n-1 \right)h}} \right]\),
\(=\underset{h\to 0}{\mathop{\lim }}\,h{{e}^{a}}\left[ \frac{{{\left( {{e}^{h}} \right)}^{n}}-1}{{{e}^{h}}-1} \right]\,\,\,\,\left[ \because a+ar+….+a{{r}^{n-1}}=a\left( \frac{{{r}^{n}}-1}{r-1} \right) \right]\),
\(=\underset{h\to 0}{\mathop{\lim }}\,h{{e}^{a}}\left( \frac{{{e}^{nh}}-1}{{{e}^{h}}-1} \right)\),
\(=\underset{h\to 0}{\mathop{\lim }}\,{{e}^{a}}\left[ \frac{\left( {{e}^{b-a}}-1 \right)}{\left( \frac{{{e}^{h}}-1}{h} \right)} \right]={{e}^{a}}\left( {{e}^{b-a}}-1 \right)={{e}^{b}}-{{e}^{a}}\).
