Geometric Interpretation of Cross Product

Geometric Interpretation of Cross Product

1. \(\vec{a}\times \vec{b}=|\vec{a}||\vec{b}|\sin \theta \hat{n}\).

\(|\vec{a}\times \vec{b}|=|\vec{a}||\vec{b}|\sin \theta \).

\(=2\left( \frac{1}{2}\times |\vec{a}||\vec{b}|\sin\theta\right)\).

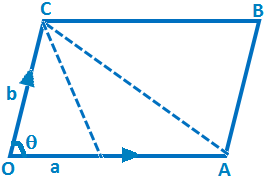
= 2 (Area of Triangle AOC)

= Area of Parallelogram

Area of the triangle OAB is \(\frac{1}{2}|\vec{a}\times \vec{b}|\).

\(\vec{a}\times \vec{b}\) is said to be the vector area of the parallelogram with adjacent sides OA and OB.

2. If \(\vec{a},\vec{b}\) are diagonals of a parallelogram, its area = \(\frac{1}{2}|\vec{a}\times \vec{b}|\).

In fig \(\overrightarrow{OC}=\overrightarrow{a}\) and \(\overrightarrow{AB}=\overrightarrow{b}\).

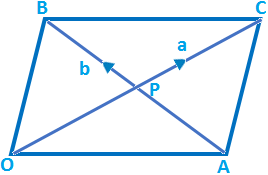
Area parallelogram = \(4\times \frac{1}{2}|\overrightarrow{PC}\times \overrightarrow{PB}|\).

= \(4\times \frac{1}{2}\left| \frac{{\vec{a}}}{2}\times \frac{{\vec{b}}}{2} \right|\).

\(=\frac{1}{2}\left| \vec{a}\times \vec{b} \right|\).

3. If AC and BD are the diagonals of a quadrilateral, then its vector area is \(\frac{1}{2}\overrightarrow{AC}\times \overrightarrow{BD}\).

Vector area of the quadrilateral ABCD = Vector area of ΔABC + Vector area of ΔACD.

\(=\frac{1}{2}\overrightarrow{AB}\times \overrightarrow{AC}+\frac{1}{2}\overrightarrow{AC}\times \overrightarrow{AD}\).

\(=-\frac{1}{2}\overrightarrow{AC}\times \overrightarrow{AB}+\frac{1}{2}\overrightarrow{AC}\times \overrightarrow{AD}\).

\(=\frac{1}{2}\overrightarrow{AC}\times (\overrightarrow{AD}-\overrightarrow{AB})\).

\(=\frac{1}{2}\overrightarrow{AC}\times \overrightarrow{BD}\).