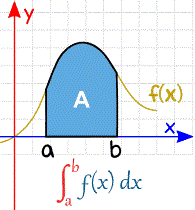
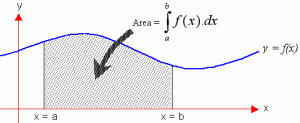
Evaluation of definite Integrals
If f(x) is a continuous function defined on [a, b] then to evaluate \(\int_{a}^{b}{f\left( x \right)}dx\), we use the following algorithm.
Algorithm:
Step I: Find a primitive or anti derivative of f(x) by computing \(\int f\left( x \right)dx\). Let this be ф(x).
Step II: Compute the values ф(x) at x = a, x = b.
Step III: Calculate ф(b) – ф(a). The number so obtained is the required value of \(\int_{a}^{b}{f\left( x \right)}dx\).
Prove: \(\int_{0}^{\pi /4}{\frac{{{x}^{2}}}{{{\left( x\sin x+\cos x \right)}^{2}}}}dx=\frac{4-\pi }{4+\pi }\).
Solution: We have
\(\int_{0}^{\pi /4}{\frac{{{x}^{2}}}{{{\left( x\sin x+\cos x \right)}^{2}}}}dx\),
\(=\int_{0}^{\pi /4}{\frac{x\cos x.x\sec x}{\left( x\sin x+\cos x \right)}}dx\),
\(=\int_{0}^{\pi /4}{\frac{d\left( x\sin x+\cos x \right)}{{{\left( x\sin x+\cos x \right)}^{2}}}}\),
\(={{\left[ x\sec x\times \frac{-1}{x\sin x+\cos x} \right]}_{0}}^{\pi /4}+\int_{0}^{\pi /4}{\frac{\left( \sec x+x\sec x\tan x \right)}{x\sin x+\cos x}}\),
\(={{\left[ \frac{-x\sec x}{x\sin x+\cos x} \right]}_{0}}^{\pi /4}+\int_{0}^{\pi /4}{{{\sec }^{2}}xdx}\),
\(={{\left[ \frac{-x\sec x}{x\sin x+\cos x} \right]}_{0}}^{\pi /4}+{{\left[ \tan x \right]}_{0}}^{\pi /4}\),
\(=\left[ \frac{-\frac{\pi }{4}\sqrt{2}}{\frac{\pi }{4\sqrt{2}}+\frac{1}{\sqrt{2}}}-0 \right]+\left( 1-0 \right)=\frac{-2\pi }{\pi +4}+1=\frac{4-\pi }{\pi +4}\).
Evaluation of define Integrals by Substitution: When the variable in a definite integral is changed, the substitution in terms of new variable should be effected at three places.
(i) In the integrated,
(ii) In the differential say dx
(iii) In the limits.
Evaluate: \(\int_{0}^{\pi /2}{\left( \sqrt{\tan x}+\sqrt{\cot x} \right)}dx\).
Solution: We have,
\(I=\int_{0}^{\pi /2}{\left( \sqrt{\tan x}+\sqrt{\cot x} \right)}dx\),
\(=\int_{0}^{\pi /2}{\frac{\sin x+\cos x}{\sqrt{\sin x\cos x}}}dx\),
\(=\sqrt{2}\int_{0}^{\pi /2}{\frac{1}{\sqrt{2\sin x\cos x}}}d\left( -\cos x+\sin x \right)\),
\(=\sqrt{2}\int_{0}^{\pi /2}{\frac{1}{\sqrt{1-{{\left( -\cos x+\sin x \right)}^{2}}}}}d\left( -\cos x+\sin x \right)\).
Let -cosx + sinx = t. Then,
x = 0 ⇒ t = -1 and \(x=\frac{\pi }{2}\) ⇒ t = 1
∴ \(I=\sqrt{2}\int_{-1}^{1}{\frac{1}{\sqrt{1-{{t}^{2}}}}}dt\),
\(=\sqrt{2}\left[ {{\sin }^{-1}}t \right]_{-1}^{1}\),
\(=\sqrt{2}\left[ {{\sin }^{-1}}1-{{\sin }^{-1}}\left( -1 \right) \right]\),
\(=\sqrt{2}\left[ \frac{\pi }{2}-\left( \frac{\pi }{2} \right) \right]\),
\(=\sqrt{2}\pi \).
