Efficiency of Carnot Cycle

A heat engine is a device that produces motion from heat and includes gasoline engines and steam engines. These devices vary in efficiency. The Carnot Cycle describes the most efficient possible heat engine, involving two isothermal processes and two adiabatic processes. It is the most efficient heat engine that is possible within the laws of physics. The efficiency of a Carnot Engine is entirely a function of the temperatures of the hot and cold reservoirs we use.

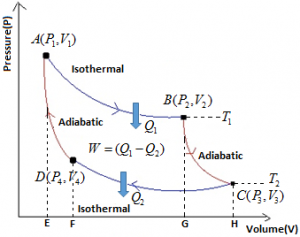
The efficiency of Engine is defined as the ratio of work done to the heat supplies. i.e.

\(\eta \,\,=\,\,\frac{Work\,\,Done}{Heat\,\,Input}\,\,=\,\,\frac{W}{{{Q}_{1}}}\).

Net Work done during complete cycle = W₁ – W₃ = Area ABCD [As W₂ = W₄]

∴ \(\eta \,\,=\,\,\frac{W}{{{Q}_{1}}}\,\,=\,\,\frac{{{W}_{1}}-{{W}_{3}}}{{{W}_{1}}}=\frac{{{Q}_{1}}-{{Q}_{2}}}{{{Q}_{1}}}=1-\frac{{{W}_{3}}}{{{W}_{1}}}=1-\frac{{{Q}_{2}}}{{{Q}_{1}}}\),

\(\eta \,\,=\,\,1-\frac{R{{T}_{2}}\,{{\log }_{e}}\left( \frac{{{V}_{3}}}{{{V}_{4}}} \right)}{R{{T}_{1}}\,{{\log }_{e}}\left( \frac{{{V}_{2}}}{{{V}_{1}}} \right)}\).

Since points B and C lie on same adiabatic curve:

∴ \({{T}_{1}}\,V_{2}^{\gamma -1}={{T}_{2}}\,V_{3}^{\gamma -1}\) (Or) \(\frac{{{T}_{1}}}{{{T}_{2}}}\,\,=\,\,{{\left( \frac{{{V}_{3}}}{{{V}_{2}}} \right)}^{\gamma -1}}\) … (1)

Also point D and A lie on the same adiabatic curve:

∴ \({{T}_{1}}V_{1}^{\gamma -1}={{T}_{2}}V_{4}^{\gamma -1}\) (Or) \(\frac{{{T}_{1}}}{{{T}_{2}}}={{\left( \frac{{{V}_{4}}}{{{V}_{1}}} \right)}^{\gamma -1}}\) … (2)

From equations (1) and (2) we get:

\(\frac{{{V}_{3}}}{{{V}_{2}}}=\frac{{{V}_{4}}}{{{V}_{1}}}\) (Or) \(\frac{{{V}_{3}}}{{{V}_{4}}}=\frac{{{V}_{2}}}{{{V}_{1}}}\),

\({{\log }_{e}}\left( \frac{{{V}_{3}}}{{{V}_{4}}} \right)\,\,=\,\,{{\log }_{e}}\left( \frac{{{V}_{2}}}{{{V}_{1}}} \right)\),

So, Efficiency of Carnot Engine, \(\eta \,\,=\,\,1-\frac{{{T}_{2}}}{{{T}_{1}}}\).