Coulomb’s Law in Vector Form
Charles Coulomb’s measured the magnitude of electric forces between charged objects using the torsion balance. From Coulomb’s experiments, we can generalize the properties of the electric force. Let, \({{q}_{1}}\) and \({{q}_{2}}\) be two like charges placed at points \(A\) and\(B\), respectively, in vacuum. \(\overrightarrow{{{r}_{1}}}\)is the position vector of point\(A\), and \(\overrightarrow{{{r}_{2}}}\) is the position vector of point\(B\). Let \(\overrightarrow{{{r}_{21}}}\) be vector from \(A\) to\(B\), then:
\(\overrightarrow{{{r}_{21}}}=\overrightarrow{{{r}_{2}}}-\overrightarrow{{{r}_{1}}}\) and \(|\overrightarrow{{{r}
_ {21}}}|=r=|\overrightarrow{{{r}
_ {2}}}-\overrightarrow{{{r}_{1}}}|\).
\(\widehat{{{r}_{21}}}=\frac{\overrightarrow{{{r}_{21}}}}{r}=\frac{\overrightarrow{{{r}_{2}}}-\overrightarrow{{{r}_{1}}}}{|\overrightarrow{{{r}_{2}}}-\overrightarrow{{{r}_{1}}}|}\).
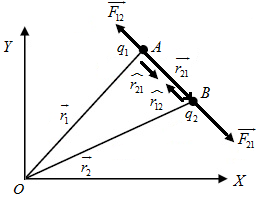
From the above figure, it is clear that \(\overrightarrow{{{F}_{21}}}\) and \(\widehat{{{r}_{12}}}\) are in the same direction, so:
\(\overrightarrow{{{F}_{21}}}=\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{{{q}_{1}}{{q}_{2}}}{{{r}^{2}}}\frac{\overrightarrow{r}}{r}=\frac{{{q}_{1}}{{q}_{2}}}{4\pi {{\varepsilon }_{0}}}\frac{\overrightarrow{r}}{{{r}^{3}}}=\frac{{{q}_{1}}{{q}_{2}}}{4\pi {{\varepsilon }_{0}}}\frac{\overrightarrow{{{r}_{1}}}-\overrightarrow{{{r}_{2}}}}{|\overrightarrow{{{r}_{2}}}-\overrightarrow{{{r}_{1}}}{{|}^{3}}}\).
The above equations give the Coulomb’s law in vector form. As we know that charges apply equal and opposite forces on each other, we have:
\(\overrightarrow{{{F}_{12}}}=-\overrightarrow{{{F}_{21}}}\) (Or) \(\overrightarrow{{{F}_{12}}}=\frac{{{q}_{1}}{{q}_{2}}}{4\pi {{\varepsilon }_{0}}}\frac{\overrightarrow{{{r}_{1}}}-\overrightarrow{{{r}_{2}}}}{|\overrightarrow{{{r}_{2}}}-\overrightarrow{{{r}_{1}}}{{|}^{3}}}\).
We can also write in terms of unit vector notation:
\(\overrightarrow{{{F}_{12}}}=\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{{{q}_{1}}{{q}_{2}}}{{{r}^{2}}}\widehat{{{r}_{12}}}\).
Where, \(\widehat{{{r}_{12}}}\)is a unit vector directed toward \({{q}_{1}}\) from\({{q}_{2}}\), this force of Coulomb’s law is illustrated in the above figure. For three different point charge distributions, we have:
\(\widehat{{{r}_{12}}}=-\widehat{{{r}_{21}}}\).
So,
\(\overrightarrow{{{F}_{12}}}=\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{{{q}_{1}}{{q}_{2}}}{{{r}^{2}}}\widehat{{{r}_{12}}}=\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{{{q}_{1}}{{q}_{2}}}{{{r}^{2}}}\left( -\widehat{{{r}_{21}}} \right)\).
\(\overrightarrow{{{F}_{12}}}=-\frac{1}{4\pi {{\varepsilon }_{0}}}\frac{{{q}_{1}}{{q}_{2}}}{{{r}^{2}}}\widehat{{{r}_{21}}}=-\overrightarrow{{{F}_{21}}}\).
