One of the fundamental conservation laws of physics is the law of conservation of angular momentum which states that the total angular momentum of a system is constant in both magnitude and direction, if the resultant external torque acting on the system is zero.
During skating, if the skater folds his or her hands then he is able to rotate at a greater speed as compares to when his/her hands are wide open. This is due to the law of Conservation of Angular Momentum.
What is Conservation of Angular Momentum: The angular momentum of a rigid object is defined as the product of the moment of inertia and the angular velocity. It is analogous to linear momentum and is subject to the fundamental constraints of the conservation of angular momentum principle. If there is no external torque on the object. Angular momentum is a vector quantity. It is derivable from the expression for the angular momentum of a particle.
Angular Momentum (L) = Moment of Inertia (I) × Angular Velocity (ω)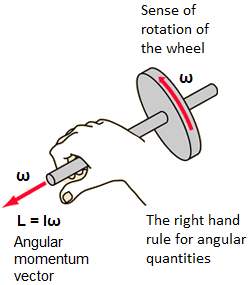
Formula to find the Conservation of Angular Momentum: The degree to which a body rotates, gives its angular momentum. It is represented by L.
Angular momentum formula is articulates as,
L = Iω
The angular velocity is ω. The moment of inertia of the rotating body about axis of rotation is I, and the angular momentum is L, when regarding linear momentum then angular momentum is articulated by
L = r x p
The linear momentum is p, the radius of the body is r from the axis crossing through centre Χ signifies the cross product.
It is articulated in Kilogram meter square per second (kg m2/s). Angular momentum formula is made use of in finding the angular momentum of the particle and also to find the parameters associated to it.
Uses of determining the Law of Conservation of Angular Momentum:
- It is used to determine the presence of a satellite with a planet.
- It is used to analyse the atom’s Bohr model.
- It is used to determine the central force motion.
- The concept of angular momentum is also useful when dealing with the orbits of satellites and planets.
How to find the Conservation of Angular Momentum?
Example: A solid cylinder of mass 500 kg rotates about its axis with angular speed of 90m/sec. If the radius of the cylinder is 0.5m. Find the angular momentum of the cylinder about its axis?
Answer: Given,
Mass (M) = 500 kg,
Angular Speed (ω) = 90 m/sec,
Radius (r) = 0.5 m,
Now,
Moment of Inertia (I) = \(\frac{m{{r}^{2}}}{2}\)
= \(\frac{500\times {{(0.5)}^{2}}}{2}\)
= 62.5 kg.m²
Now, we know that:
Angular Momentum (L) = Iω
= 62.5 x 90
Angular Momentum (L) = 5625 kg.m²/sec