Collinear and Coplanar Vectors
Collinear Vectors: They can have equal or unequal magnitudes and their directions may be same or opposite. Two vectors are collinear if they have the same direction or are parallel or anti-parallel. They can be expressed in the form a = k b where a and b are vectors and ‘ k ‘ is a scalar quantity. If \(\overrightarrow{a}\), \(\overrightarrow{b}\) are any two non – collinear vectors and x, y are scalars, then \(x\overrightarrow{a}\,+\,y\overrightarrow{b}\,=\,\overrightarrow{0}\,\Rightarrow \,x\,=\,y\,=\,0\).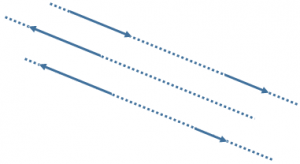
Example: if \(\overrightarrow{a}\) and \(\overrightarrow{b}\) are non-collinear vectors, find the value of x for which the vectors \(\overrightarrow{\alpha }\,=\,\left( 2x\,+\,1 \right)\overrightarrow{a}\,-\,\overrightarrow{b}\) and \(\overrightarrow{\beta }\,=\,\left( x\,-\,2 \right)\overrightarrow{a}\,+\,\overrightarrow{b}\) are collinear.
Solution: Vectors \(\overrightarrow{\alpha }\) and \(\overrightarrow{\beta }\) will be collinear, if \(\overrightarrow{\alpha }\,=\,m\overrightarrow{\beta }\) for some scalar m.
\(\left( 2x\,+\,1 \right)\overrightarrow{a}\,-\,\overrightarrow{b}\,=\,m\left[ \left( x\,-\,2 \right)\overrightarrow{a}\,+\,\overrightarrow{b} \right]\),
\(\left[ \left( 2x\,+\,1 \right)\,-\,m\left( x\,-\,2 \right) \right]\overrightarrow{a}\,-\,\left( m\,+\,1 \right)\overrightarrow{b}\,=\,\overrightarrow{0}\),
\(\left( 2x\,+\,1 \right)\,-\,m\left( x\,-\,2 \right)\,=\,0\) and \(-\left( m\,+\,1 \right)\,=\,0\),
\(m\,=\,-\,1\) and \(x\,=\,\frac{1}{3}\).
Coplanar Vectors: A system of vectors is said to be coplanar, if their supports are parallel to the same plane.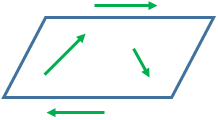
Let \(\overrightarrow{a}\) and \(\overrightarrow{b}\) be two given non-zero non-collinear vectors. Then any vectors \(\overrightarrow{r}\) coplanar with \(\overrightarrow{a}\) and \(\overrightarrow{b}\) can be uniquely expressed as \(\overrightarrow{r}\,=\,x\overrightarrow{a}\,+\,y\overrightarrow{b}\) for some scalars x and y.
Example: Show that the vectors\(\overrightarrow{a}\,-\,2\overrightarrow{b}\,+\,3\overrightarrow{c}\), \(\overrightarrow{a}\,-\,3\overrightarrow{b}\,+\,5\overrightarrow{c}\)and \(-\,2\overrightarrow{a}\,+\,3\overrightarrow{b}\,-\,4\overrightarrow{c}\) are coplanar.
Solution:
\(A\,=\,\overrightarrow{a}\,-\,2\overrightarrow{b}\,+\,3\overrightarrow{c}\),
\(B\,=\,\overrightarrow{a}\,-\,3\overrightarrow{b}\,+\,5\overrightarrow{c}\),
\(C\,=\,-2\overrightarrow{a}\,+\,3\overrightarrow{b}\,-\,4\overrightarrow{c}\).
\(\bar{A}.\left[ \overline{B}\times \overline{C} \right]=\left|\begin{matrix}1 & -2 & 3 \\1 & -3 & 5 \\-2 & 3 & -4 \\\end{matrix} \right|\).
1 (12 – 15) + 2 (- 4 + 10) + 3 (3 – 6)
– 3 + 12 – 9 = 0
Hence, the given vectors are coplanar.