Centroid of Triangle & Centroid of Tetrahedron

Centroid of Triangle: The coordinates of the centroid of the triangle with vertices A (x₁, y₁, z₁) B (x₂, y₂, z₂) and C (x₃, y₃, z₃)

\(\left( \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3},\,\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}}{3},\,\frac{{{z}_{1}}+{{z}_{2}}+{{z}_{3}}}{3} \right)\)

Proof: Let D be the mid-point of AC then coordinates of D are \(\left( \frac{{{x}_{2}}+{{x}_{3}}}{2},\,\frac{{{y}_{2}}+{{y}_{3}}}{2},\,\frac{{{z}_{2}}+{{z}_{3}}}{2} \right)\)

Let G be the centroid of ΔABC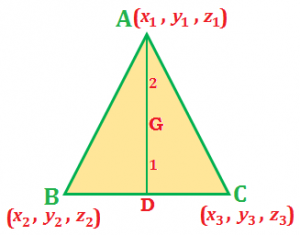Then, G divides AD in the ratio 2:1

So, coordinate of D are \(\left( \frac{1.{{x}_{1}}+2\left( \frac{{{x}_{2}}+{{x}_{3}}}{2} \right)}{1+2},\,\frac{1.{{y}_{1}}+2\left( \frac{{{y}_{2}}+{{y}_{3}}}{2} \right)}{1+2},\,\frac{1.{{z}_{1}}+2\left( \frac{{{z}_{2}}+{{z}_{3}}}{2} \right)}{1+2} \right)\)

i.e, \(\left( \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3},\,\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}}{3},\,\frac{{{z}_{1}}+{{z}_{2}}+{{z}_{3}}}{3} \right)\)

Centroid of Tetrahedron: Let ABCD be a tetrahedron such that the coordinates of its vertices are A (x₁, y₁, z₁) B (x₂, y₂, z₂) C (x₃, y₃, z₃) and D (x₄, y₄, z₄) the coordinates of the centroid of faces ABC, DAB, DBC and DCA are respectively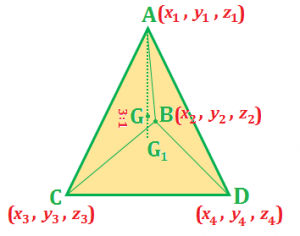\({{G}_{1}}=\left[ \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3},\,\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}}{3},\,\frac{{{z}_{1}}+{{z}_{2}}+{{z}_{3}}}{3} \right]\),

\({{G}_{2}}=\left[ \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{4}}}{3},\,\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{4}}}{3},\,\frac{{{z}_{1}}+{{z}_{2}}+{{z}_{4}}}{3} \right]\),

\({{G}_{3}}=\left[ \frac{{{x}_{2}}+{{x}_{3}}+{{x}_{4}}}{3},\,\frac{{{y}_{2}}+{{y}_{3}}+{{y}_{4}}}{3},\,\frac{{{z}_{2}}+{{z}_{3}}+{{z}_{4}}}{3} \right]\),

\({{G}_{4}}=\left[ \frac{{{x}_{4}}+{{x}_{3}}+{{x}_{1}}}{3},\,\frac{{{y}_{4}}+{{y}_{3}}+{{y}_{1}}}{3},\,\frac{{{z}_{4}}+{{z}_{3}}+{{z}_{1}}}{3} \right]\).

Now, coordinates of point G dividing DG, in the ratio 3:1 are

\(\left[ \frac{1.{{x}_{4}}+3\left( \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3} \right)}{1+3},\,\frac{1.{{y}_{4}}+3\left( \frac{{{y}_{1}}+{{y}_{2}}+{{y}_{4}}}{3} \right)}{1+3},\,\frac{1.{{z}_{4}}+3\left( \frac{{{z}_{2}}+{{z}_{3}}+{{z}_{4}}}{3} \right)}{1+3} \right]\),

= \(\left[ \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}+{{x}_{4}}}{4},\,\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}+{{y}_{4}}}{4},\,\frac{{{z}_{1}}+{{z}_{2}}+{{z}_{3}}+{{z}_{4}}}{4} \right]\),

Similarly the point dividing CG₁, CG₂, AG₃ and BG₄ in the ratio 3:1 has the same coordinates.

Hence, the point \(G\left[ \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}+{{x}_{4}}}{4},\,\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}+{{y}_{4}}}{4},\,\frac{{{z}_{1}}+{{z}_{2}}+{{z}_{3}}+{{z}_{4}}}{4} \right]\).