Centripetal Force
When an object travels in a circle, even at constant speed, it is undergoing acceleration. In this case the acceleration acts not to increase or decrease the magnitude of the velocity vector, but rather to change its direction. Newton’s second Law tells us that in the absence of any outside Force, an object will travel at constant speed in a straight line. Therefore if we observe the direction of motion changing, we know there is a Force acting. The direction of that Force is the direction that the velocity vectors turns toward. In the case of circular motion, we can see that the acceleration in inward since the direction of motion is forever turning inward toward the centre.
What is Centripetal Force?
If an object accelerates according to the changes of velocity, then it can change either its speed or direction of motion. In simple terms, if any moving object in a circular path is constantly changing its direction means it is constantly accelerating.
Using the Newton’s second law of motion, if an object is travelling in a circular path, it is found that the centripetal force of an object moving in a circular path always acts towards the centre of the circle.
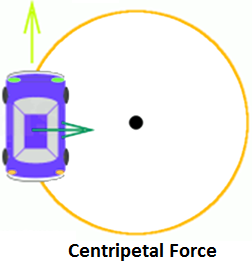
Formula of Centripetal Force:
Centripetal Force (Fp) = mv²/r
Where,
Fp = Centripetal Force
ap = Centripetal Acceleration
m = Mass
v = Speed
r = radius.
