Centroid: The centroid of a triangle is the point of intersection of medians. It divides medians in 2: 1 ratio.
If A(x1, y1), B(x2, y2), C(x3, y3) are vertices of triangle ABC, then coordinates of centroid is \(G=\left( \frac{{{x}_{1}}+{{x}_{2}}+{{x}_{3}}}{3},\,\frac{{{y}_{1}}+{{y}_{2}}+{{y}_{3}}}{3} \right)\).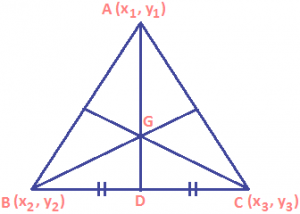
In center: Point of intersection of angular bisectors
Coordinates of \(I=\left( \frac{a{{x}_{1}}+b{{x}_{2}}+c{{x}_{3}}}{a+b+c},\,\frac{a{{y}_{1}}+b{{y}_{2}}+c{{y}_{3}}}{a+b+c} \right)\).
Where a, b, c are sides of triangle ABC.
Circumcentre: Point of intersection of perpendicular bisectors.
Co-ordinates of circumcentre O is \(O=\left( \frac{{{x}_{1}}\sin 2A+{{x}_{2}}\sin 2B+{{x}_{3}}\sin 2C}{\sin 2A+\sin 2B+\sin 2C},\,\frac{{{y}_{1}}\sin 2A+{{y}_{2}}\sin 2B+{{y}_{3}}\sin 2C}{\sin 2A+\sin 2B+\sin 2C} \right)\).
Orthocenter: Point of intersection of altitudes of triangle ABC.
Coordinates of orthocenter H is \(H=\left( \frac{{{x}_{1}}\tan A+{{x}_{2}}\tan B+{{x}_{3}}\tan C}{\tan A+\tan B+\tan C},\,\frac{{{y}_{1}}\tan A+{{y}_{2}}\tan B+{{y}_{3}}\tan C}{\tan A+\tan B+\tan C} \right)\).
Note:
- Orthocenter of a right angled triangle is at its vertex forming the right angle.
- The orthocenter H, circumcentre O and centroid G of a triangle are collinear and G divides H, O in ratio 2 : 1 i.e., HG : OG = 2: 1
- Circumcentre of a right angled triangle is mid-point of hypotenuse.
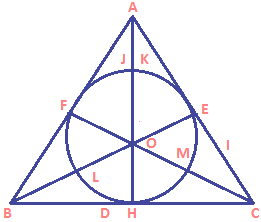
Nine Point Circle: Let ABC be triangle such that AD, BE and CF are its altitudes, H, I, J are midpoints of line segments of sides BC, CA, AB respectively; K, L, M are midpoints of joining orthocenter (O) to angular points A, B, C. These 9 points (D, E, F, H, I, J, K, L, M) are concyclic and the circle passing through nine points is nine point circle and Center is known as nine- point center.