Spherical Capacitor

Spherical Capacitor

A spherical capacitor consists of two concentric spherical conducting shells of radii \(a\) and \(b\), say \(b>a\). The outer shell is earthed. Place a charge \(+Q\) on the inner shell. It will reside on the outer surface of the shell. A charge \(-Q\) will be induced on the inner surface of the outer shell. A charge \(+Q\) will flow from the outer shell to earth.

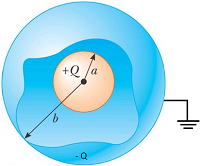
Consider a Gaussian spherical surface of radius \(r\) such that \(a<r<b\). Form Gauss’s law, the electric field at distance \(r>a\) is \(E=\frac{Q}{4\pi {{\varepsilon }_{0}}{{r}^{2}}}\).

The potential difference is: \({{V}_{b}}-{{V}_{a}}=-\int\limits_{a}^{b}{\overrightarrow{E}.\overrightarrow{dr}}=-\int\limits_{a}^{b}{\frac{Q}{4\pi {{\varepsilon }_{0}}{{r}^{2}}}}dr\).

Since, \({{V}_{b}}=0\) we have:

\({{V}_{a}}=\int\limits_{a}^{b}{\frac{Q}{4\pi {{\varepsilon }_{0}}{{r}^{2}}}}dr=\frac{Q}{4\pi {{\varepsilon }_{0}}}\left( \frac{1}{a}-\frac{1}{b} \right)=\frac{Q\left( b-a \right)}{4\pi {{\varepsilon }_{0}}ab}\).

Now, the capacitance is: \(C=\frac{Q}{{{V}_{a}}-{{V}_{b}}}=\frac{Q}{{{V}_{a}}}=\frac{4\pi {{\varepsilon }_{0}}ab}{\left( b-a \right)}\).

Therefore, the capacitance of a spherical capacitor is: \(C=\frac{4\pi {{\varepsilon }_{0}}ab}{\left( b-a \right)}\).