Point of Contact – Circles
1. The condition that the straight-line lx + my + n = 0 may touch the circle x² + y² = a² is n² = a² (l² + m²) and the point of contact is \(\left( \frac{-{{a}^{2}}l}{n},\,\frac{-{{a}^{2}}m}{n} \right)\).
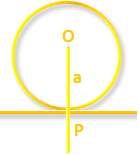
Proof: Given line is lx + my + n = 0 … (1)
Given circle is x² + y² = a² … (2)
Centre O (0, 0) and radius = a
Line (1) touches circle (2)
⟺ the perpendicular distance from O to line (1) is \(a=\left| \frac{l(0)+m(0)+n}{\sqrt{{{l}^{2}}+{{m}^{2}}}} \right|\) \(\left( \because \,\,d=\left| \frac{ax+by+c}{\sqrt{{{a}^{2}}+{{b}^{2}}}} \right| \right)\),
⟺ \(\left| \frac{n}{\sqrt{{{l}^{2}}+{{m}^{2}}}} \right|=a\),
n² = a² (l² + m²)
Let P (x₁, y₁) be the point of contact.
The equation of the tangent to the circle (1) at P is xx₁ + yy₁ – a² = 0 … (3)
Now (1) and (3) represent the same line
∴ \(\frac{{{x}_{1}}}{l}=\frac{{{y}_{1}}}{m}=-\frac{{{a}^{2}}}{n}\),
\(\Rightarrow {{x}_{1}}=-\frac{{{a}^{2}}l}{n},\,\,{{y}_{1}}=-\frac{{{a}^{2}}m}{n}\) ,
Point of contact\(=\left( \frac{-{{a}^{2}}l}{n},\,\frac{-{{a}^{2}}m}{n} \right)\).
2. The condition for the straight line lx + my + n = – may be a tangent to the circle x² + y² + 2gx + 2fy + c = 0 is (g² + f² – c) (l² + m²) = (lg + mf -n)².
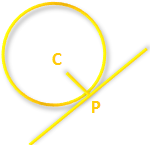
Proof: The given line is lx + my + n = 0 … (1)
The given circle is x² + y² + 2gx + 2fy + c = 0 … (2)
Centre C = (-g, -f),
radius r = √g² + f² – c
Line (1) is a tangent to the circle (2)
⟺ The perpendicular distance from the center C to the line (1) is equal to the radius r
∵ \(\sqrt{{{g}^{2}}+{{f}^{2}}-c}=\left| \frac{ax+by+c}{\sqrt{{{a}^{2}}+{{b}^{2}}}} \right|\),
⟺ \(\left| \frac{-\lg -mf+n}{\sqrt{{{l}^{2}}+{{m}^{2}}}} \right|=\sqrt{{{g}^{2}}+{{f}^{2}}-c}\) (∵ The perpendicular distance equal to radius of the circle).
⟺ (lg + mf – n)² = (g² + f² – c) (l² + m²)
The condition for the straight-line y = mx + c to touch the circle x² + y² = a² is c² = a² (l + m²).
3. If the straight-line y = mx + c touches the circle x² + y² = a², then their point of contact is \(\left( -\frac{{{a}^{2}}m}{c},\,\frac{{{a}^{2}}}{c} \right)\).
Proof: Let P (x₁, y₁) be the point of contact
Given line is y = mx + c
⟺ mx – y + c = 0 … (1)
The equation of tangent to the circle x² + y² = a² at P is xx₁ + yy₁ – a² = 0 … (2)
Now (1) and (2) represent the same line.
\(\frac{{{x}_{1}}}{m}=\frac{{{y}_{1}}}{-1}=\frac{-{{a}^{2}}}{c}\),
⇒ \({{x}_{1}}=\frac{-{{a}^{2}}m}{c},\,\,{{y}_{1}}=\frac{{{a}^{2}}}{c}\),
∴ Point of contact\(=\left( \frac{-{{a}^{2}}m}{c},\,\frac{{{a}^{2}}}{c} \right)\).
