Pair of Tangents – Circles

Pair of Tangents – Circles

The equation to the pair of tangents to the circle S = 0 from P (x₁, y₁) is S²₁ = S₁₁S.

Let the equation of the circle be S ≡ x² + y² + 2gx + 2fy + c = 0

Let a line L = 0 through P (x₁, y₁) meets the circle in A and B.

Let Q (x, y) be any point on the line

Let k : 1 be the ratio in which A divides PQ

∴ \(A=\left( \frac{kx+{{x}_{1}}}{k+1},\,\frac{ky+{{y}_{1}}}{k+1} \right)\).

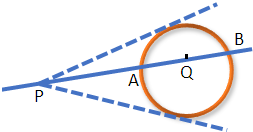
Since A is a point on the circle, it follows that

\({{\left( \frac{kx+{{x}_{1}}}{k+1} \right)}^{2}}+{{\left( \frac{ky+{{y}_{1}}}{k+1} \right)}^{2}}+2g\left( \frac{kx+{{x}_{1}}}{k+1} \right)+2f\left( \frac{ky+{{y}_{1}}}{k+1} \right)+c=0\),

⇒ (kx + x₁)² + (ky + y₁)² + 2g (kx + x₁) (k + 1) + 2f (ky + y₁) (k+1) + c (k+1)² = 0

⇒ k² [x² + y² + 2gx + 2fy + c] + 2k [xx₁ + yy₁ + g (x + x₁) + f (y + y₁) + c] + [x²₁ + y²₁ + 2gx₁ + 2fy₁ + c] = 0

⇒ k²S + 2kS₁ + S₁₁ = 0 … (1)

If L = 0 is a tangent to S = 0, then A and B are coinciding and the roots of (1) are equal.

∴ (2S₁)² = 4S₁₁ S → S²₁ = S₁₁ S.

∴ The locus of Q is S²₁ = S₁₁ S.

∴ The equation to the pair of tangents from (x₁, y₁) is S²₁ = S₁₁S.

Example: Find the equation to the pair of tangents drawn from (4, 10) to the circle x² + y² = 25.

Solution: Given that,

The equation of the pair of tangents drawn from (4, 10) to x² + y² = 25 is

The equation to the pair of tangents from (x₁, y₁) is S²₁ = S₁₁S.

S²₁ = (xx₁ +y y₁ – 25)² = (4x₁ + 10y₁ – 25)²

S₁₁ = x²₁ + y₁² – 25 at point (4, 10)

S₁₁ = x²₁ + y₁² – 25 = (16 + 100 – 25)

(x₁ + 10y₁ – 25)² = (16 + 100 – 25) (x² + y² – 25)

16x² + 80xy + 100y² – 200x – 500y + 625 = 91 (x² + y² + y² – 25)

 75x² – 80xy – 9y² + 200x + 500y – 2900 = 0.