Maxima and Minima
Maximum: Let f (x) be function with domain D ⊂ R then f (x) is said to attain the maximum value at a point a ϵ D if f (x) ≤ f (a) ∀ x ϵ D. The point is called the point maximum f (a) is known as Maximum value of f(x).
Minimum: Let f(x) be a function with Domain D ⊂ R. Then f(x) is said to attain the minimum value at a point a ϵ D f (x) ≥ f (a) ∀ x ϵ D.
The point a is called the point minimum f (a) is known as Minimum value of f (x)
Local Maxima: A function f (x) is said to attain a local maximum at x = a if ∃ a neighborhood (a – δ, a + δ) of a such that f (x) ≥ f (a) ∀ x ϵ (a – δ, a + δ), x ≠ 0
In such a case f (a) is called the local maximum value of f (x) at x = a. Local maximum value of f(x) at x = a
Local Minima: A function f(x) is said to attain a local minimum at x = a if ∃ a neighborhood (a – δ, a + δ) of a such that f (x) > f (a) ∀ x ϵ (a – δ, a + δ), x ≠ 0 then f (a) is called local minimum of f (x) at x = 0.
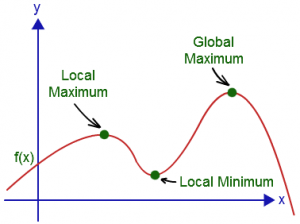
The points at which a function attains either the local maximum values or local minimum values are known as extreme points and both local maximum and minimum values are called the extreme values of f (x) at an extreme values of f (x).
At an extreme values of f (x). At an extreme point ‘a’, f (x) – f (a) keeps the same sign ∀x is in a deleted neighborhood of a
Example: Find the point at which the local maxima or local minima are attained for the following function. Also find the local maxima or local minimum value f (x) = x³ – 3x
Solution: f(x) = x³ – 3x
Differentiation with respect to the x
f’(x) = 3x² – 3 and again apply differentiation with respect to the x
f‘’ (x) = 6x – 0
f‘’ (x) = 6x
For maximum or minimum f‘(x) = 0
f’(x) = 3x² – 3 = 0
3x² – 3 = 0
x = ± 1
f‘’(1) = 6(1) = 6 > 0
Therefore f(x) has minimum at x = 1 and that minimum value is f (1) = (1)³ – 3(1) = -2
f’’(-1) = (-1) 6 = – 6 < 0
Therefore f(x) has maximum value at x = -1 and that maximum value is f (-1) = (-1)³ – 3(-1) = -1 + 3 = 2.
